dsp.SampleRateConverter
Multistage sample rate converter
Description
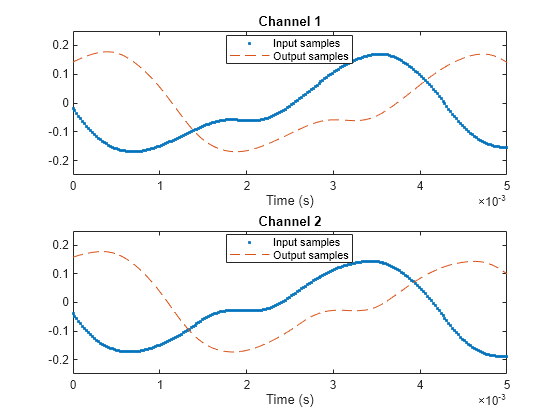
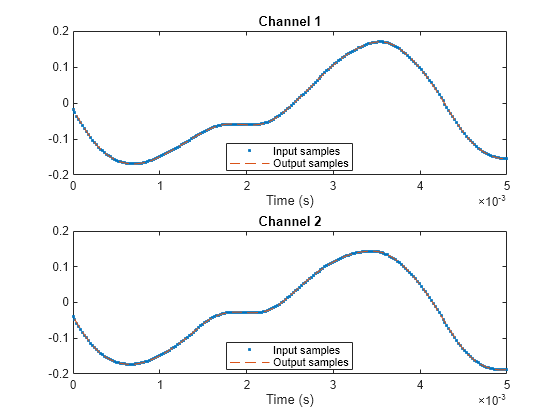
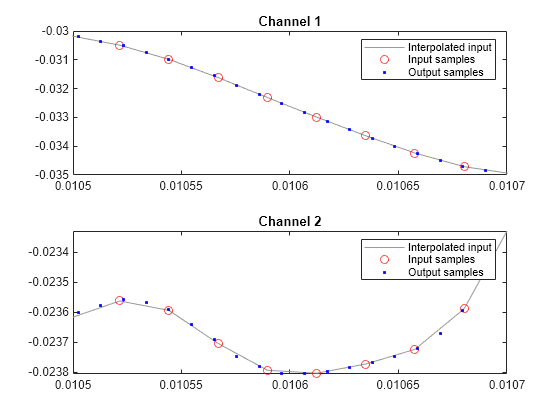
The SampleRateConverter
System object™ converts the sample rate of an incoming signal.
To convert the sample rate of a signal:
Create the
dsp.SampleRateConverterobject and set its properties.Call the object with arguments, as if it were a function.
To learn more about how System objects work, see What Are System Objects?
This object supports C/C++ code generation and SIMD code generation under certain conditions. For more information, see Code Generation.
Creation
Description
src = dsp.SampleRateConvertersrc, that converts the sample rate of each channel of
an input signal.
src = dsp.SampleRateConverter(Name=Value)src, with properties and options specified by one or
more Name-Value pair arguments.
Properties
Usage
Syntax
Description
Input Arguments
Output Arguments
Object Functions
To use an object function, specify the
System object as the first input argument. For
example, to release system resources of a System object named obj, use
this syntax:
release(obj)
Examples
Algorithms
The general multistage sample rate converter performs a multistage decimation, a single-stage sample rate conversion, and a multistage interpolation. Actual designs include at most two of these steps.
The procedure automatically determines the optimal number of decimation or interpolation stages. In special cases, the algorithm can perform a decimation or interpolation in a single stage.
The algorithm always attempts to start by reducing the sample rate. This decreases the amount of computation required. The decimation step ensures that the intermediate sample rate does not go below the bandwidth of interest and that the block does not filter out any information.
Each individual stage uses halfband or Nyquist filters to minimize the number of nonzero coefficients.
The algorithm allows transition-band aliasing because it decreases the implementation cost. It keeps the signal within the bandwidth of interest alias free up to the value specified by the
StopbandAttenuationproperty.
Extended Capabilities
Version History
Introduced in R2014bSee Also
Functions
cost|freqz|getActualOutputRate|getFilters|info|visualize|getRateChangeFactors