미국 인구 예측(Predicting the US Population)

이 예제에서는 차수가 높은 다항식을 사용하여 데이터를 외삽하는 것이 위험하고 신뢰할 수 없음을 보여줍니다.

이 예제는 MATLAB®이 출시되기 이전부터 사용되었습니다. 이 예제는 1977년에 Prentice-Hall에서 출판한 Computer Methods for Mathematical Computations(저자: Forsythe, Malcolm, Moler)에 연습 과제로 처음 소개되었습니다.

이제는 MATLAB을 통해 파라미터에 변화를 주고 결과를 확인하는 것이 훨씬 더 쉬워졌지만 기본적인 수학 원리는 변하지 않았습니다.

1910년부터 2000년까지의 미국 인구 조사 데이터로 2개의 벡터를 만들고 플로팅해 보겠습니다.

% Time interval
t = (1910:10:2000)';

% Population
p = [91.972 105.711 123.203 131.669 150.697...
    179.323 203.212 226.505 249.633 281.422]';

% Plot
plot(t,p,'bo');
axis([1910 2020 0 400]);
title('Population of the US 1910-2000');
ylabel('Millions');

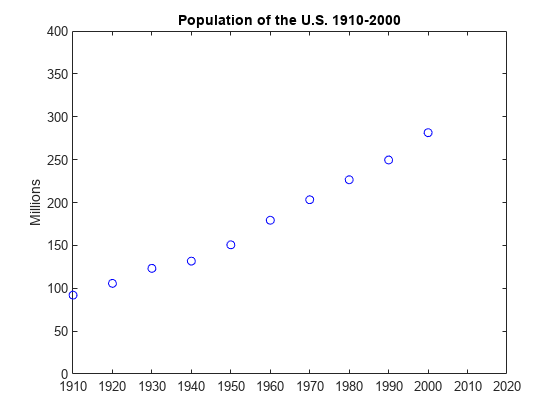
Figure contains an axes object. The axes object with title Population of the US 1910-2000, ylabel Millions contains a line object which displays its values using only markers.

2010년도의 인구는 어느 정도나 될 것으로 추측됩니까?

t에 관한 다항식으로 데이터를 피팅하고 이를 사용하여 t = 2010일 때 인구를 외삽합니다. 11×11 크기의 방데르몽드 행렬을 포함하는 선형 연립방정식을 풀어 다항식의 계수를 구합니다. 이 행렬의 요소는 A(i,j) = s(i)^(n-j)로서, 스케일링된 시간의 거듭제곱입니다.

n = length(t);
s = (t-1950)/50;
A = zeros(n);
A(:,end) = 1;
for j = n-1:-1:1
   A(:,j) = s .* A(:,j+1);
end

방데르몽드 행렬의 마지막 d+1개 열을 포함하는 다음 선형 연립방정식을 풀어 데이터 p를 피팅하는, 차수가 d인 다항식의 계수 c를 구합니다.

A(:,n-d:n)*c ~= p

d < 10이면, 미지수보다 방정식이 더 많은 경우가 되므로 최소제곱해가 적절합니다.
d == 10이면, 방정식의 완전한 해를 구할 수 있으며 다항식은 실제로 데이터를 보간합니다.

두 경우 모두 백슬래시 연산자를 사용하여 시스템을 풀 수 있습니다. 다음은 3차 피팅의 계수입니다.

c = A(:,n-3:n)\p

이제 1910년부터 2010년까지 매년 다항식을 계산하고 결과를 플로팅해 보겠습니다.

v = (1910:2020)';
x = (v-1950)/50;
w = (2010-1950)/50;
y = polyval(c,x);
z = polyval(c,w);

hold on
plot(v,y,'k-');
plot(2010,z,'ks');
text(2010,z+15,num2str(z));
hold off

Figure contains an axes object. The axes object with title Population of the US 1910-2000, ylabel Millions contains 4 objects of type line, text. One or more of the lines displays its values using only markers

3차 피팅과 4차 피팅을 비교해 보면 외삽 지점이 매우 다른 것을 알 수 있습니다.

c = A(:,n-4:n)\p;
y = polyval(c,x);
z = polyval(c,w);

hold on
plot(v,y,'k-');
plot(2010,z,'ks');
text(2010,z-15,num2str(z));
hold off

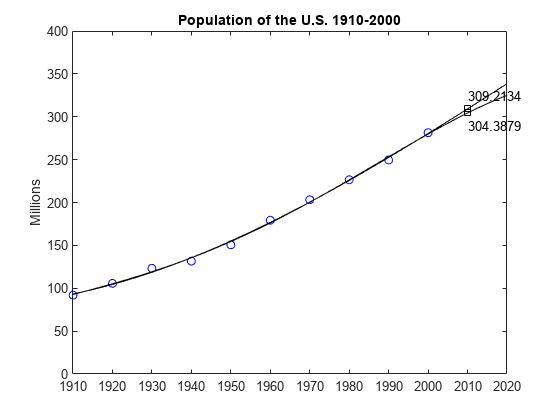
Figure contains an axes object. The axes object with title Population of the US 1910-2000, ylabel Millions contains 7 objects of type line, text. One or more of the lines displays its values using only markers

차수가 증가할수록 외삽의 오차가 오히려 커집니다.

cla
plot(t,p,'bo')
hold on
axis([1910 2020 0 400])
colors = hsv(8);
labels = {'data'};
for d = 1:8
   [Q,R] = qr(A(:,n-d:n));
   R = R(1:d+1,:);
   Q = Q(:,1:d+1);
   c = R\(Q'*p);    % Same as c = A(:,n-d:n)\p;
   y = polyval(c,x);
   z = polyval(c,11);
   plot(v,y,'color',colors(d,:));
   labels{end+1} = ['degree = ' int2str(d)];
end
legend(labels, 'Location', 'NorthWest')
hold off

Figure contains an axes object. The axes object contains 9 objects of type line. One or more of the lines displays its values using only markers These objects represent data, degree = 1, degree = 2, degree = 3, degree = 4, degree = 5, degree = 6, degree = 7, degree = 8.

참고 항목

polyfit