GREATCIRCLE and LOXODROME: "As the crow flies" and rhumb lin
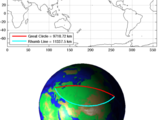
The function GREATCIRCLE computes the shortest path along the great circle ("as the crow flies") between two points defined by their geographic coordinates (latitude and longitude). With one output argument it returns distance or vector of distances, with two or more output arguments it returns path coordinates and optional vector of distances and bearing angles along the path.
The function LOXODROME computes the path with a constant bearing, crossing all meridians of longitude at the same angle. It returns also a vector of distances and the bearing angle.
Loxodrome path (also known as "rhumb line") is longer than great circle one, but still used in navigation as it is easier to follow with a compass.
Type "doc greatcircle" or "doc loxodrome" for syntax, help and examples.
인용 양식
François Beauducel (2024). GREATCIRCLE and LOXODROME: "As the crow flies" and rhumb lin (https://www.mathworks.com/matlabcentral/fileexchange/37652-greatcircle-and-loxodrome-as-the-crow-flies-and-rhumb-lin), MATLAB Central File Exchange. 검색됨 .
MATLAB 릴리스 호환 정보
플랫폼 호환성
Windows macOS Linux카테고리
- Mathematics and Optimization > Mapping Toolbox > Geometric Geodesy >
- Radar > Mapping Toolbox > Geometric Geodesy >
- Sciences > Earth, Ocean, and Atmospheric Sciences > Earth and Planetary Science >
태그
도움
줌: pathdist
Community Treasure Hunt
Find the treasures in MATLAB Central and discover how the community can help you!
Start Hunting!| 버전 | 게시됨 | 릴리스 정보 | |
|---|---|---|---|
| 1.4.0.0 | - greatcircle: fixes the singularity when LON1 and LON2 have 180° of difference (thanks to Patrick Hew's comment) |
||
| 1.3.0.0 | - greatcircle: two possible syntaxes (output distances or path way)
|
||
| 1.2.0.0 | BUG CORRECTION: bearing angle (and distances) are now with correct values. |
||
| 1.1.0.0 | - add loxodrome function
|
||
| 1.0.0.0 |